2024.9.04
Chemistry at pirika.com > Chemistry > Chemical engineering > Reprint: gas-liquid equilibrium estimation by ASOG. > Chapter 1: Basic equations of solution theory > Chapter 2: ASOG method. > 2.3 Actual ASOG method calculation
2.3.5 Assessment of liquid-liquid equilibrium using the ASOG method
The 1986 book uses the same akl as the 1979 book but with various extensions. Various ASOG families have been developed, including high-pressure gas-liquid equilibrium, liquid-liquid equilibrium and solid-liquid equilibrium, and their programmes are described. ASOG for vapour-phase association systems is also a separate programme. For some families, a different parameter has been developed than the group interaction parameter akl for low-pressure vapour-liquid equilibrium.
Although there are parameters for ASOG-LL to calculate liquid-liquid equilibrium,
For the groups CH2,OH,H2O, the group Wilson parameters at 25 °C have only been determined as follows. Since the temperature is constant at 25°C, there is only one parameter.
aCH2/H2O=0.2858 aH2O/CH2=0.000585
aCH2/OH=0.1704 aOH/CH2=0.0127
aH2O/OH=1.5865 aOH/H2O=0.581
This leaves only water, alcohol and saturated hydrocarbons to calculate. Nor is it expected to increase in the future.
Here, as one of the applications, we will consider the octanol/water partition ratio using the low-pressure vapour-liquid equilibrium akl.
Let us also consider the solubility in water, which is important in this context.
If it is a medicine, we think of a liquid-liquid extraction of a certain compound as a medicine.
Two immiscible solvents are used as the liquid-liquid at that time. Water/hexane, water/diethyl ether, water/chloroform, etc.
For example, if the raw material is water-soluble and the medicine made from it is oil-soluble, if it is dissolved in water/diethyl ether, the unreacted raw material will dissolve in the water phase and the medicine in the diethyl ether. Therefore, they can be easily separated using a separating funnel.
Among these liquid-liquid extraction systems, the octanol/water partition ratio (logKow) is a special system. Octanol dissolves in water and water dissolves in octanol. This is complicated by the fact that the third component also partitions into it. However, a very large number of systems are available as experimental values. The reason for this is said to be that the polarity of octanol is close to that of living organisms, and the degree to which drugs and other substances are taken into the body can be easily correlated with logKow. The logKow has been measured for a great number of compounds.
Let’s first estimate and look at octanol/water liquid-liquid extraction of alcohol compounds, as there is a lot of data and it is of high importance.
Use 1979 for the MN parameter.
When solvent pairs are selected, two different pairs are displayed for one alcohol: water and 1-Octanol.
First select (1) water/Ethanol. Next, open the VLE Calc. and you will see the following screen.
The parameters of the chosen pair are displayed in the AiSOG Result. Here, the DB values for the solubility in water, octanol/water partition ratio, Henry’s constant and molecular volume of the second molecule, Ethanol, are displayed. These values are noted down.
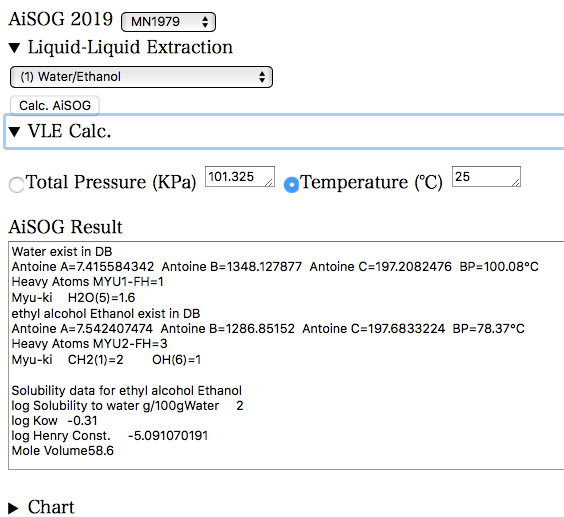
Here, the calculation is based on a constant temperature of 25 °C. If the conditions are correct, press the Calc. AiSOG button.
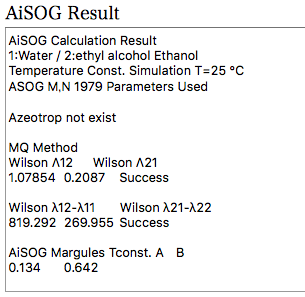
When the calculation is finished, the result is added.
Repeat for all solvent pairs, noting down the values, although it is a little harder.
(Use a spreadsheet software for the rest of the process.)
The following table is then created.
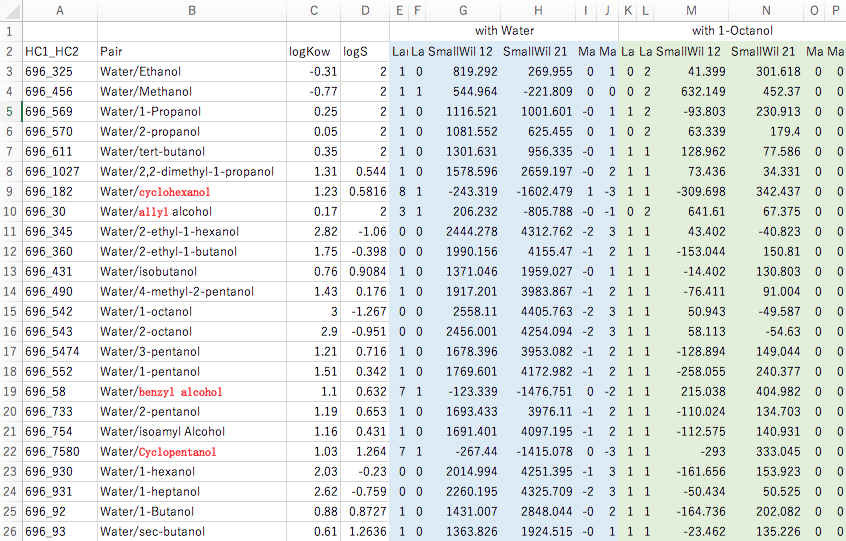
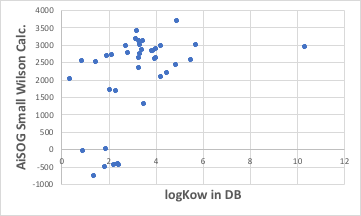
Using the ASOG method to estimate the vapour-liquid equilibrium at a constant temperature of 25°C, one pair each of Large Wilson, Small Wilson and Magules parameters can be obtained. Since there is one for water and one for 1-Octanol, 12 parameters are determined for one alcohol. For example, the log Kow for ethanol is -0.31, which can be examined graphically to see which parameters it is correlated with.
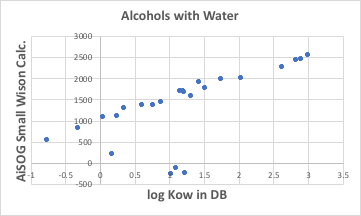
It can then be seen that there is a very high correlation with the Small Wilson12 parameter with water.
There are solvents that deviate significantly from the line at about four points. These would be cyclic alcohols, alcohols with unsaturated bonds (double bonds) and benzyl alcohol, as in MN1979 the C=C, ArCH and CyCH groups do not have the interglue interaction parameter akl with H2O determined. (Benzyl alcohol has another factor, which will be discussed later.)
Once such a correlation is found, the octanol/water liquid-liquid extraction equilibrium constants for the chain alcohols can be obtained by simply calculating the Small Wilson12 parameter with water in ASOG. It is just a matter of fitting it to this graph.
This is strange when you think about it.
Octanol plays no role.
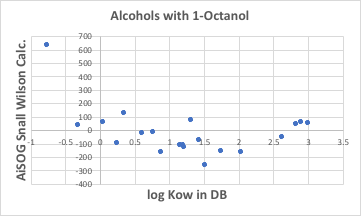
In fact, if we graph the Small Wilson12 parameters for octanol and the other alcohols, there is almost no correlation, as shown in the following graph.
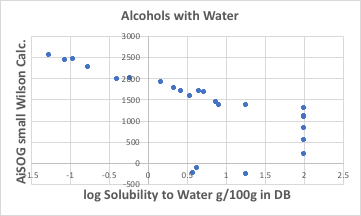
So what is the solubility in water? As expected, there is a very high correlation with Small Wison12. (Note that water solubility data can vary from g/100g water, mg/L, mol/L. In many dissolution tests, logS≥2 is meaningless because the test is terminated as free mixing when 100g is dissolved in 100g water.)
Dissolution in water is also a dominant factor for Small Wilson12, which means that logKow is also dominated by Small Wilson12. In fact, it is known that there is a very high correlation between logKow and logS.
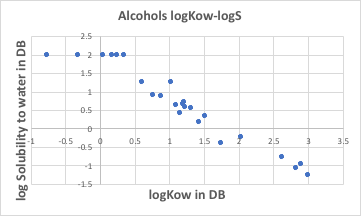
This is very strange, as solubility in water is a matter of headroom. Solubility in water is a matter of quantity; logKow is a matter of ratio. Whether solubility in octanol/solubility in water is 100/100 or 0.1/0.1, logKow is zero. There is such a correlation between quantity and ratio. Let me elaborate on this point a little more.
For a rough calculation, if the solubility in water is Sw and the solubility in octanol is So, the relationship between the two is as follows, based on the relationship in the previous graph.
log(So/Sw)=logKow=-logSw+2
logSo-logSw=-logSw+2
logSo=2.
This means that any solute will dissolve in octanol at 100 g/100 g octanol. Let’s look again at the graph of Small Wilson12 parameters for octanol and solutes. With the exception of methanol on the left-hand side of the diagram, the Small Wilson12 values fall between -300 and 200. This is a very small value compared to Small Wilson12 with water and solute.
This means that the total amount that was not dissolved in water is allocated to octanol in the alcohol system.
So what happens when the solute is an ester compound? As with alcohols, there is a very high correlation between Small Wilson12 and logKow with water. However, Small Wilson12 is larger than for alcohols. (They are less soluble.)
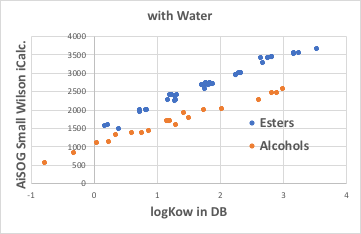
Small Wilson12 with octanol is larger than with alcohols. Even so, it can be said that the total amount that was not dissolved in water is allocated to octanol, which is the same as for alcohols.
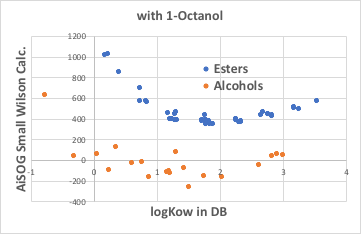
The relationship between logKow and logS does not differ significantly between esters and alcohols.
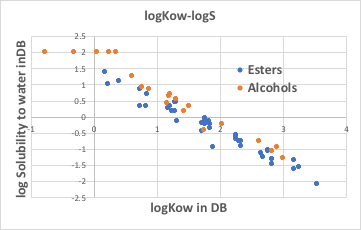
What we have found is that the octanol/water liquid-liquid extraction equilibrium constant is highly correlated with Small Wilson12 with water for each family. Once the slope and intercept of each line are assigned to a family, logKow can be estimated from only the Small Wilson12 with water calculated by ASOG. However, Small Wilson12 with octanol may come into play as the solubility in octanol decreases.
It is in some ways correct and in some ways incorrect to say that logKow is a ratio value and logS is a quantity value. Since the dominant factor is Small Wilson12 with water, logKow is a quantity value, as is solubility in water. Whether the distribution is 1000/1000, 1/1 or 0.001/0.001, logKow = log(1) = 0. However, logKow originally only looks at 0.001/0.001 because the solute dissolved in the measurement is only very thin, dilute solution.
So why are these activity coefficients not used to estimate liquid-liquid extraction equilibrium constants?
The answer is probably simple: because even a single drug is evaluated by attaching various groups to the basic skeleton, so that the various families are mixed up and the correlation with Small Wilson12 with water is obscured.
If this happens, big data analysis is a good thing: AI will no longer use activity coefficients as variables.
As mentioned earlier, logKow has relatively big data. And logKow is correlated with the Bio Concentration Factor (BCF).
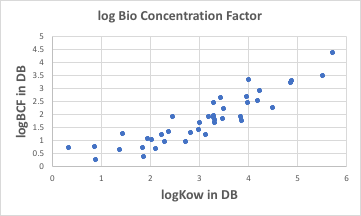
This log(BCF) is also in mg/Kg, so it is easy to mistake it for a ratio, but it is a matter of quantity because it is a value per Kg of body weight. The reason it is highly correlated with logKow is that logKow is also essentially a quantity.
All compounds with known logKow are subjected to machine learning using AI by calculating the molecular orbitals and the topology of the molecule. The contents are a black box, but you find an answer that seems to be somehow correct. Such research is increasing. This is not where chemists come in. But we don’t usually calculate molecular orbitals for interactions with water or octanol. Such calculations are still very difficult.
Small Wilson12
(λ(water/Solute)-λ(water/water) ), where λ(water/Solute) represents the interaction energy between different molecules.
That can be calculated using the ASOG method, so there is no need to bother with molecular orbital calculations. Machine learning, in which the AI learns indicators that indicate that and the family, is a unique way for chemists to proceed.
If we want to estimate logBCF, a measure of bioconcentration, we should question the treatment of octanol as representative of biopolarity.
Biological fats and oils are mainly composed of glycol esters. If ethyl octanoate (EPA:C7H15COOC2H5) is used instead of octanol, it is easy to calculate how much it might change from octanol.
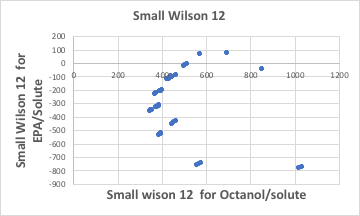
Thus, the interaction energy between different molecules for an alcohol compound (octanol) and that for an ester compound (EPA) behave quite differently. The only difference is that the correlation between logKow and logBCF will vary.
Only chemists who have been confronted with the interaction energies between different molecules could provide such information to the AI.
Such information would be essential for research in biochemical engineering.
Copyright pirika.com since 1999-
Mail: yamahiroXpirika.com (Replace X with @)
The subject line of the email should start with [pirika].
