2024.9.04
Chemistry at pirika.com > Chemistry > Chemical engineering > Reprint: gas-liquid equilibrium estimation by ASOG. > Chapter 1: Basic equations of solution theory > Chapter 2: ASOG method. > 2.3 Actual ASOG method calculation
2.3.2 Heterogeneous system
In systems with a large deviation from the ideal solution, e.g. n-butanol-water and methyl ethyl ketone-water systems, the liquids do not mix with each other. The liquid phase then splits into two phases, resulting in a gas-liquid equilibrium. In such systems, the fugacity concept described in 1.6 Basic equations for vapour-liquid equilibrium is required.
fiV (Fugacity of component i in the gas phase.)
=fiI (I Fugacity of component i in liquid phase)
=fiII (II Fugacity of component i in the liquid phase) i=1,2, …, N (2-7)
where superscript V denotes the gas phase, I and II the two liquid phases, liquid phase I and liquid phase II.
Recall the definition of fugacity. Fugacity is ‘the tendency to escape from a phase’ and ‘in equilibrium they are balanced’. The following equation then holds.
fiV = Pyi =γiI xiI PiS = γiII xiII PiS i=1,2, …, N (2-8)
xiI , xiII are the mole fractions of component i in liquid phases I and II, γiI , γiII are the activity factors of component i in liquid phases I and II.
Simply put, the ‘tendency of the i-component to escape from the I-liquid phase’ is equal to the ‘tendency of the i-component to escape from the II-liquid phase’ and, furthermore, to the ‘tendency of the i-component to escape from the gas phase’.
There is a Gibbs phase rule. It describes the relationship between the number of components and phases and the degrees of freedom of state quantities (temperature, pressure) when equilibrium is reached.
F(degree of freedom)=C(Number of components)-P(Number of phases)+2
For example, in a one-component vapour-liquid equilibrium, the degrees of freedom = 1 (component) – 2 (vapour-liquid phase) + 2 = 1.
So, if the temperature is determined, the pressure is determined, and if the pressure is determined, the temperature is determined. One degree of freedom means that only one can be freely changed.
In a two-component vapour-liquid equilibrium, the degree of freedom is 2. Once the mole fraction, which is one of the state quantities, and the temperature or pressure are determined, the rest is determined.
If the liquid phase splits into two phases, the degrees of freedom = 2 (components) – 3 (vapour-liquid phase) + 2 = 1.
In other words, once the mole fraction is determined, the temperature and pressure are also determined.
Check with the Methyl Ethyl Ketone – Water system to understand the meaning of Gibbs’ phase law.
(70) Select the Methyl Ethyl Ketone (MEK)_Water system and perform the calculations in MN 1979.
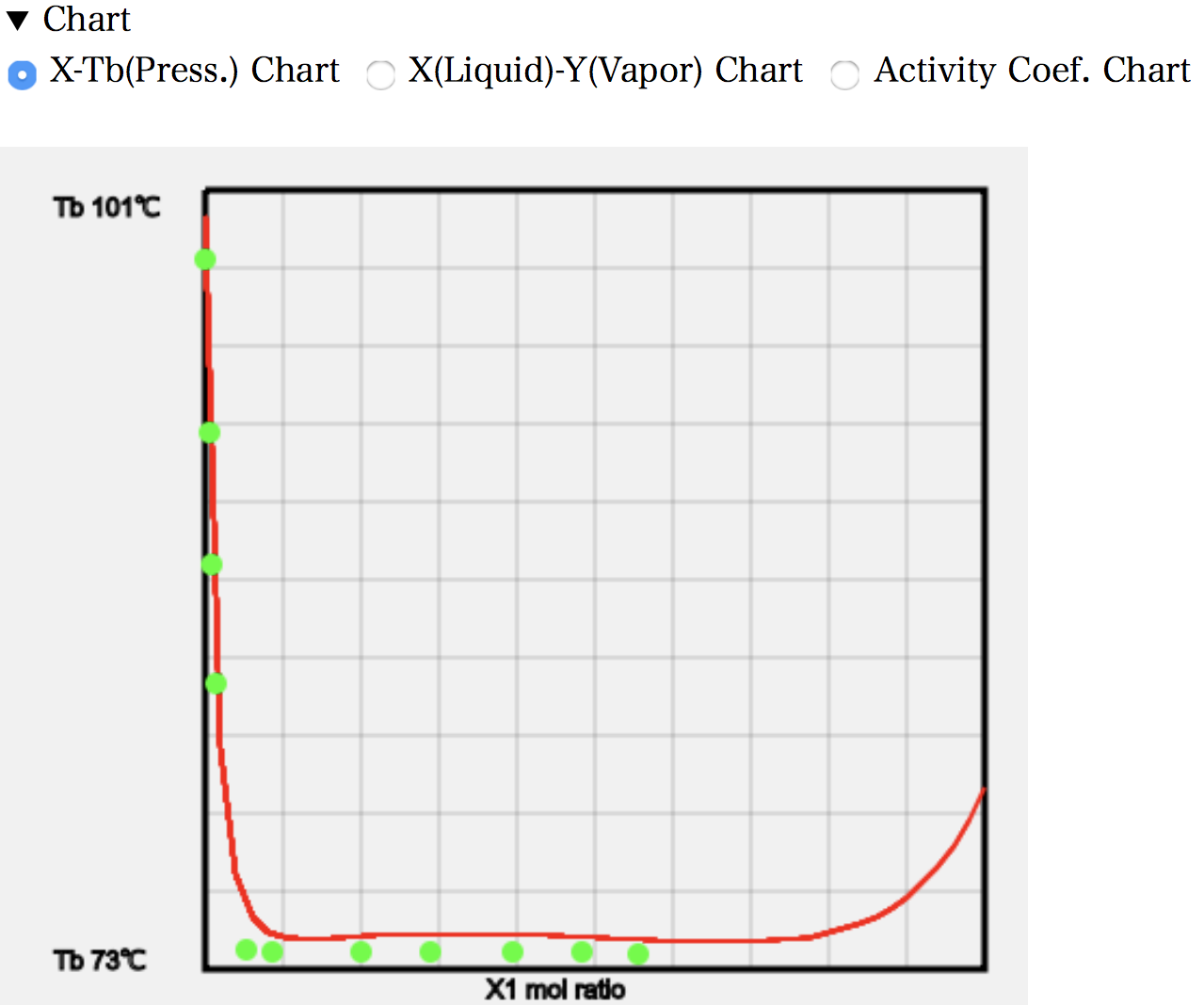
The X-T diagram shows that as the amount of MEK increases (left-hand side of the diagram), the boiling point rapidly decreases. This indicates a rapid loss of hydrogen bonds in the water. The temperature then remains unchanged over a wide range. This measurement is a constant pressure experiment. In other words, it is a system with one degree of freedom, where the temperature and pressure are both determined when the mole fraction is determined.
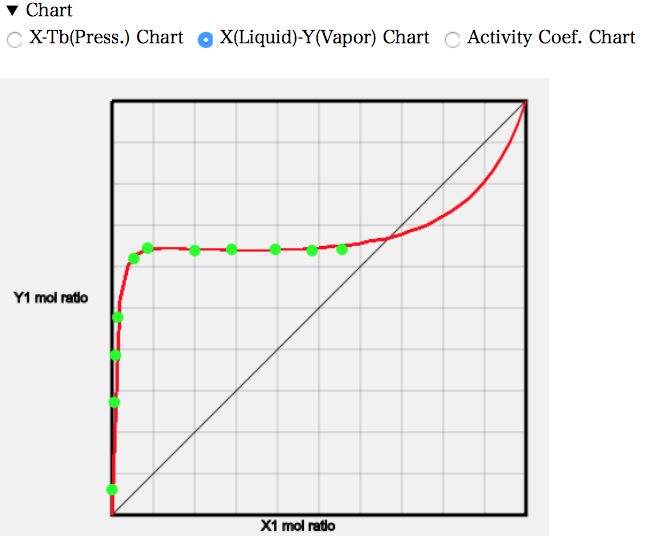
The X-Y diagram shows that the mole fraction of MEK in the gas phase is almost constant from about 0.08.
22.3 g of MEK dissolves in 100 g of water at 25 °C. The molecular weight of water is 18 and that of MEK is 72.1, so the mole fraction is 0.053. The higher temperature means that a little more will dissolve and droplets of MEK will be generated from a mole fraction of around 0.08. If MEK is increased beyond that, the MEK/water ratio in the first liquid phase will remain unchanged at 0.08, and the MEK phase in the second liquid phase will only increase. So, even if the mole fraction of MEK increases, the mole fraction of MEK in the gas phase will not increase.
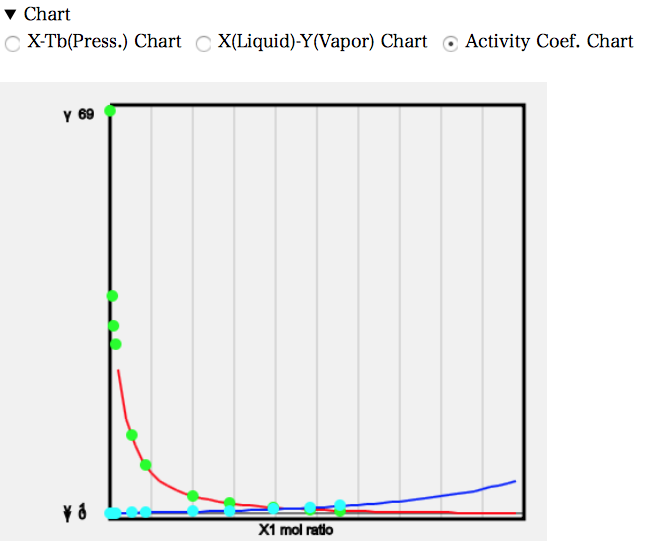
In practice, the solubility of water in MEK is also non-zero, so the activity coefficient equation for heterogeneous systems obtained from the fugacity is followed. The chart of the activity coefficient is then as follows.
It can be seen that the activity coefficient of MEK is very large at low concentrations. In general, solvent pairs with activity coefficients above 10 are said to be immiscible; the Wilson activity coefficient equation used in the ASOG method, which is said to be applicable only to homogeneous systems, is quite good.
In this way, the vapour-liquid equilibrium can be calculated simply by specifying two solvent pairs. An important feature of the ASOG method is that the results can be used to determine the state of mixing.
Copyright pirika.com since 1999-
Mail: yamahiroXpirika.com (Replace X with @.)
The subject line of the email should start with [pirika].
