2024.9.04
Chemistry at pirika.com > Chemstry > Chemical engineering > Reprint: gas-liquid equilibrium estimation by ASOG. > Chapter 1: Basic equations of solution theory
1.4 Calculation of activity coefficients
To determine the activity coefficient γ1 γ2 for a given solvent pair, experimental data on the composition and boiling point of the vapour phase are required, with the concentration of the liquid phase being varied slightly. For example, experimental data for the ethanol/water vapour-liquid equilibrium can be obtained in the form of the following table, where X1 is the fraction of ethanol in the liquid phase and Y1 is the fraction of ethanol in the gas phase.
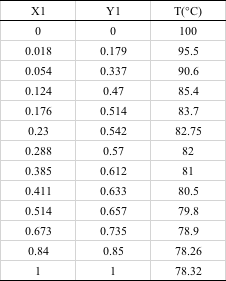
The data in this table are illustrated in two ways: the first is called the X-Y diagram, where X is the mole fraction of the liquid phase of the first component and Y is the mole fraction of the gas phase of the first component.
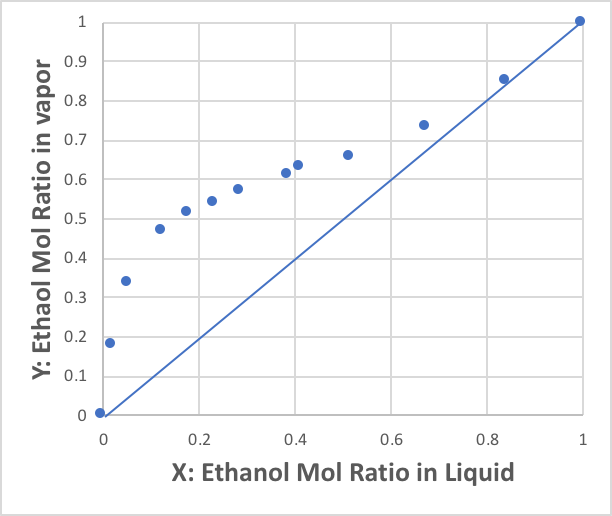
It clearly shows that the ethanol wants to pop out into the gas phase.
The second is called the X-T diagram. The abscissa is the same mole fraction of the liquid phase of the first component as in the X-Y diagram, but Y represents the boiling point.
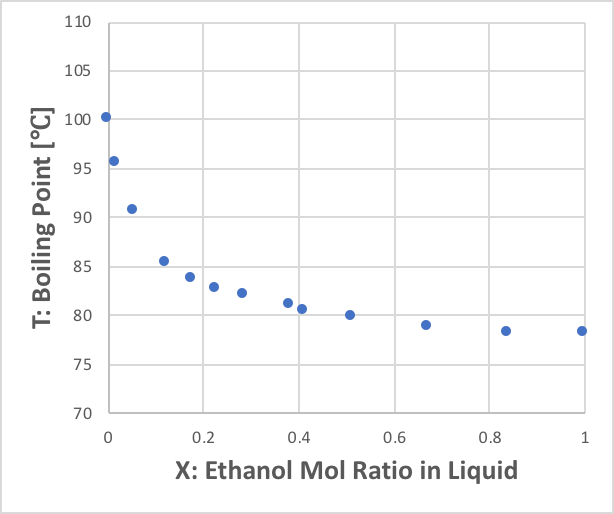
It can be seen that the boiling point falls rapidly as the mole fraction of ethanol increases. This indicates that the network of hydrogen bonds in water is broken by the addition of ethanol, allowing water molecules to jump out of the liquid phase into the gas phase even at low temperatures.
では、次に、実際に活量係数を求めてみよう。
表の最初と最後の行は純成分なので計算する必要はない。2行目を見るとこの分率では95.5℃で沸騰するとある。95.5℃でのエタノールの蒸気圧は、1446.9mmHg, 水の蒸気圧は645.7mmHgになる。
に当てはめると、
になる。
Let us now actually find the activity coefficient γ1 γ2.
The first and last rows of the table are pure components, so there is no need to calculate them; looking at the second row, it says that this fraction boils at 95.5 °C. The vapour pressure of ethanol at 95.5 °C is 1446.9 mmHg and that of water is 645.7 mmHg.
Applying P=P1*γ1*x1+P2*γ2*x2,
which results in,
760=1446.9*γ1*0.018+645.7*γ2*0.982
Also, since the gas phase composition fraction Y1 at that time is 0.179,
0.179=1446.9*γ1*0.018/760 is established.
So, γ1 and γ2 at each fraction can be easily calculated. The results are displayed graphically as follows. In the situation where ethanol is mixed with only a small amount of water (left-hand side of the diagram), the activity coefficient of water is almost 1, but the activity coefficient of ethanol is more than 5. This means that ethanol molecules surrounded by water do not want to stay there. Essentially, at a temperature of 95.5°C, it has a vapour pressure of 1446.9 mmHg, indicating that it can escape into the gas phase more than five times that amount.
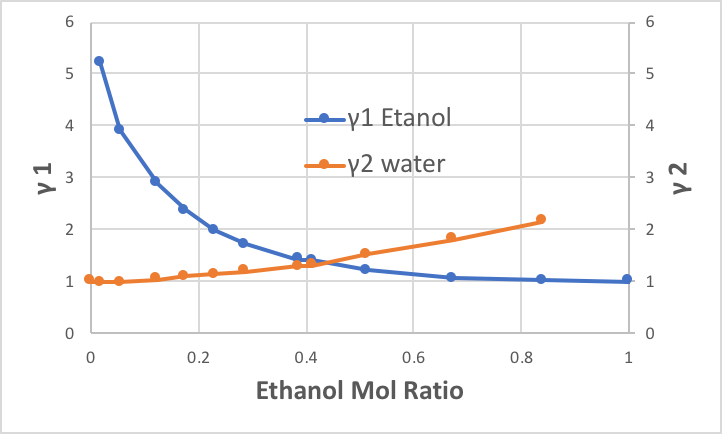
Conversely, on the right-hand side of the diagram, the activity coefficient of ethanol is 1, but the activity coefficient of water does not become much larger. This indicates that water is not so uncomfortable when surrounded by ethanol.
As experimental values, values can be read from these charts even if the exact value, e.g. x1=0.5, has not been determined.
Next section: 1.5 Coefficient of activity equation
Copyright pirika.com since 1999-
Mail: yamahiroXpirika.com (Replace X with @.)
The subject line of the email should start with [pirika].
